Analysis of Deformation and Breakage During Bubble Collision

doi:10.19596/j.cnki.1001-246x.8363

Abstract

Abstract:

Gas-liquid two-phase flow is used to simulate double bubble collision process numerically with LES method, fluid volume method, crushing criterion and the third-generation vortex identification method. The single variable method is used to study the influence of bubble diameter ratio, relative eccentricity and relative distance between bubbles on the degree of bubble fragmentation. In the process of double bubble collision, the closer the diameter of the two bubbles is, the weaker the bubble breaking degree is. The relative eccentric distance has little effect on the bubble breaking degree. It shows that as the relative distance is less than 1, the bubble breaking degree is more obvious with the increase of the relative distance. As the relative distance is more than 1, the bubble breaking degree tends to be flat. It also shows that the third-generation vortex identification method captures the vortex position in the two-phase turbulent flow field well, and reflects sensitively the turbulent changes.

Key words: bubble, aspect ratio, reduction ratio, numerical simulation, the third-generation vortex identification

Tengfei ZHAO, Hua ZHANG. Analysis of Deformation and Breakage During Bubble Collision[J]. Chinese Journal of Computational Physics, 2022, 39(1): 41-52.

Figures/Tables 18

Fig.1 A schematic of vortex and bubble size in crushing process

Fig.2 A schematic of bubbles collision

Fig.3 Contrast of bubble shape fitting (a)experimental image; (b)fitting image

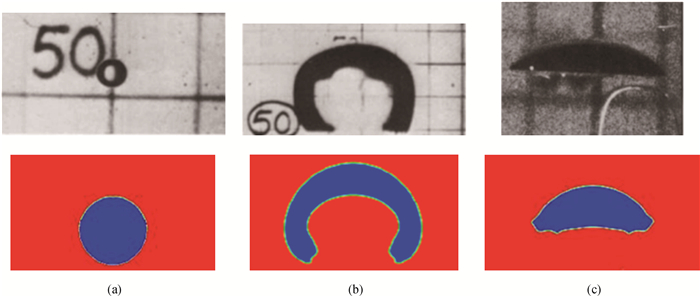
Fig.4 Contrast of single bubble ascending motion in still liquid at different moments(The upper row are images of experiment; The lower row are images of simulation.) (a) t=0.01 s; (b) t=0.1 s; (c) t=0.22 s

Table 1 Positive center collision calculation examples

算例序号	先导气泡直径/mm	尾随气泡直径/mm	直径比	相对距离ξ_z
1	2	8	0.25	0.75
2	4	8	0.5	0.75
3	6	8	0.75	0.75
4	8	8	1	0.75
5	8	6.4	1.25	0.75
6	8	5.3	1.5	0.75
7	8	4.57	1.75	0.75
8	8	4	2	0.75
9	8	3	2.67	0.75
10	8	3	2	0.25, 0.5, 0.75, 1

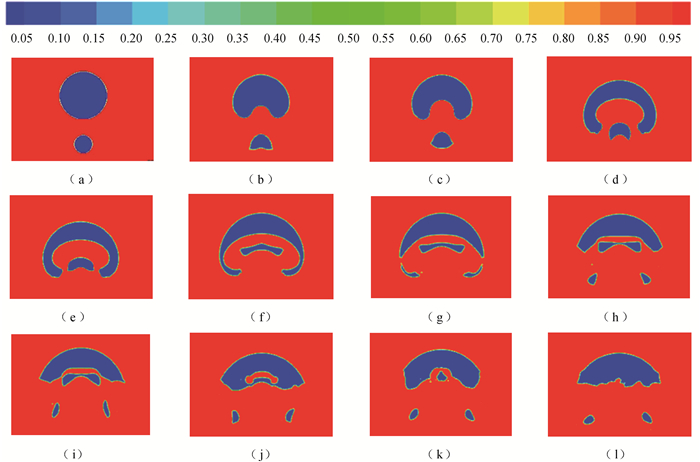
Fig.5 Shape evolution in rising process of positive center collision of two bubbles (a) t=0 s; (b) t=0.04 s; (c) t=0.05 s; (d) t=0.08 s; (e) t=0.09 s; (f) t=0.11 s; (g) t=0.12 s; (h) t=0.14s; (i) t=0.15 s; (j) t=0.16 s; (k) t=0.17 s; (l) t=0.19 s

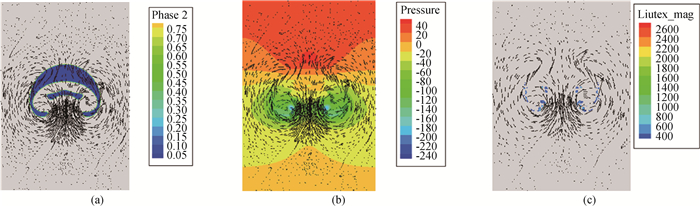
Fig.6 Bubble motion in positive center collision at 0.11 second (a) gas phase and velocity vectors; (b) pressure and velocity vectors; (c) Liutex vector and velocity vectors

Fig.7 Evolution of aspect ratios before bubbles coalesce

Fig.8 Reduction ratio as a function of relative bubble distance

Fig.9 Evolution of aspect ratios as the diameter ratio is 0.25

Fig.10 Evolution of aspect ratio as the diameter ratio is 2

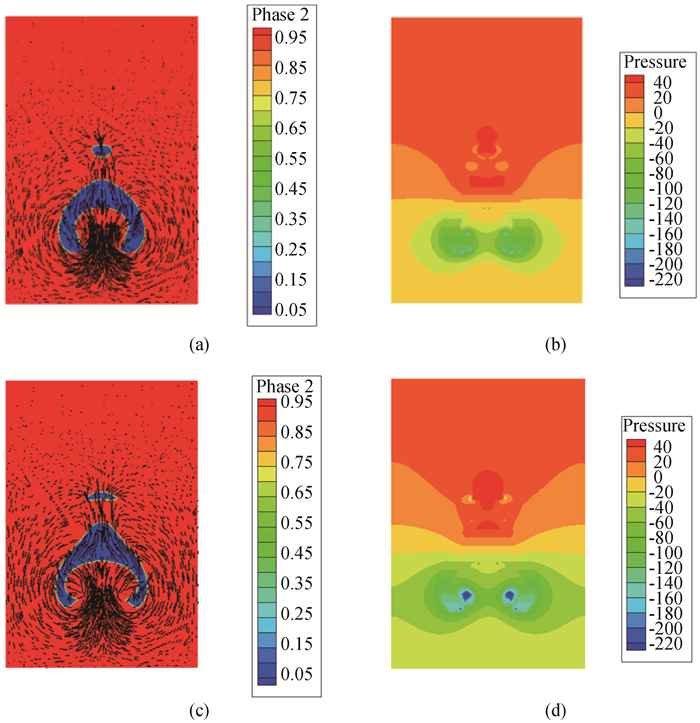
Fig.11 Velocity vectors and pressure cloud maps during bubble rising (a) velocity vectors at 0.09 s; (b) pressures at 0.09 s; (c) velocity vectos at 0.11 s; (d) pressures at 0.11 s

Fig.12 Crushing ratio as a function of diameter ratio

Fig.13 Shape evolution in rising process of eccentric collision of two bubbles (a) t=0 s; (b) t=0.04 s; (c) t=0.06 s; (d) t=0.09 s; (e) t=0.1 s; (f) t=0.11 s; (g) t=0.12 s; (h) t=0.13 s; (i) t=0.14 s; (g) t=0.16 s; (k) t=0.17 s; (l) t=0.19 s

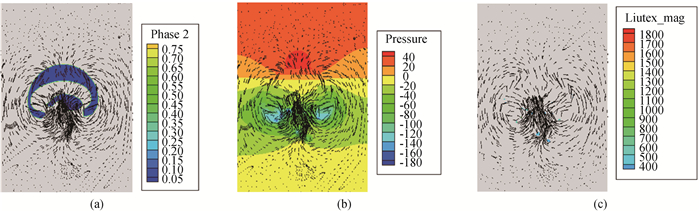
Fig.14 Bubble motion in eccentric collision at 0.1 second (a) gasous phase and velocity vectors; (b) pressure and velocity vectors; (c) Liutex vectors and velocity vectors

Fig.15 Evolution of aspect ratio and major axis slope as relative eccentricity is 0.25

Fig.16 Evolution of aspect ratio and major axis slope as relative eccentricity is 0.75

Fig.17 Crushing ratio as a function of relative eccentricity

References 35

1	RUBIO J , SOUZA M L , SMITH R W . Overview of flotation as a wastewater treatment technique[J]. Minerals Engineering, 2002, 15 (3): 139- 155. DOI
2	WANG Ziang , YU Bin , HANG Haotian , et al. Numerical study on influence of reaction mechanisms on reactive shock bubble interaction[J]. Chinese Journal of Computational Physics, 2018, 35 (4): 388- 396.
3	WANG S , ZHENG L , HAN X , et al. Lipid accumulation and CO₂ utilization of two marine oil-rich microalgal strains in response to CO₂ aeration[J]. Acta Oceanologica Sinica, 2018, 37 (2): 119- 126. DOI
4	XU L , WANG F , GUO C , et al. Improved algal oil production from Botryococcus braunii by feeding nitrate and phosphate in an airlift bioreactor[J]. Engineering in Life Sciences, 2012, 12 (2): 171- 177. DOI
5	PARIHAR R U K . Production of bio-hydrogen gas from wastewater by anaerobic fermentation process: A review[J]. International Journal of Chemical Studies, 2015, 3 (3): 07- 14.
6	QU X , SUI H , LIU Y , et al. An experimental study on two-phase characteristics of vertical injection of gas through a slot nozzle into a liquid bath[J]. Experimental Thermal and Fluid Science, 2020, 120 (2021): 1102- 1122.
7	RUIZ-RUS J , BOLAÑOS-JIMÉNEZ R , SEVILLA A , et al. Bubble pressure requirements to control the bubbling process in forced co-axial air-water jets[J]. International Journal of Multiphase Flow, 2020, 133 (20): 1034- 1067.
8	RUIZ-RUS J , BOLAÑOS-JIMÉNEZ R , SEVILLA A , et al. Bubble formation regimes in forced co-axial air-water jets[J]. International Journal of Multiphase Flow, 2020, 128 (12): 1032- 1096.
9	张阿漫, 王诗平, 白兆宏, 等. 不同环境下气泡脉动特性实验研究[J]. 力学学报, 2011, 43 (1): 71- 83.
10	张阿漫, 姚熊亮, 李佳. 气泡群的动态物理特性研究[J]. 物理学报, 2008, 57 (3): 1672- 1682.
11	ZHANG K , HOU Y D , TIAN W X , et al. Theoretical prediction of single bubble motion in vertically upward two-phase flow across inclined tube bundles[J]. Annals of Nuclear Energy, 2019, 128 (2019): 422- 432.
12	LI L J , ZHANG L , ZHU W B , et al. Simulation of a pair of bubbles rising side by side using VOSET method[J]. Chinese Journal of Computational Physics, 2015, 32 (5): 545- 52.
13	LV Yaqi , NIE Deming , LIN Jianzhong . Lattice Boltzmann simulation of gas bubble merging in three dimensions[J]. Chinese Journal of Computational Physics, 2015, 32 (5): 553- 560.
14	戚定满, 鲁传敬, 何友声. 两空泡运动特性研究[J]. 力学季刊, 2000, 21 (1): 16- 20.
15	MOORE E , CORRALES L R , DESAI T , et al. Molecular dynamics simulation of Xe bubble nucleation in nanocrystalline UO₂ nuclear fuel[J]. Journal of Nuclear Materials, 2011, 419 (1/3): 140- 144.
16	MAXIM K . Cosmological probes for supersymmetry[J]. Symmetry, 2015, 7 (2): 815- 842. DOI
17	闻昭权. 气泡聚并机理的实验及模型研究[D]. 湘潭: 湘潭大学, 2019.
18	严鹏. 鼓泡塔内气液流动行为的CFD-PBM耦合模型数值模拟[D]. 北京: 北京石油化工学院, 2019.
19	LI S , KOU J , SUN C . Effect of bubble surface properties on bubble-particle collision efficiency in froth flotation[J]. Minerals, 2020, 10 (4): 36- 54.
20	李帅, 孙龙泉, 张阿漫. 水中上浮气泡动态特性研究[J]. 物理学报, 2014, 63 (18): 291- 303.
21	孙姣, 姜楠, 陈文义, 等. 静止水中单个近壁大气泡运动特性的实验研究[J]. 实验力学, 2019, 34 (6): 973- 980.
22	李文强, 焦守华, 唐珂, 等. 静水中单气泡运动特性实验研究[J]. 原子能科学技术, 2020, 54 (9): 1652- 1659.
23	LUO H , SVENDSEN H F . Theoretical model for drop and bubble breakup in turbulent dispersions[J]. AIChE Journal, 1996, 42 (5): 1225- 1233. DOI
24	LIU Q , LUO Z . Modeling bubble column reactor with the volume of fluid approach: Comparison of surface tension models[J]. Chinese Journal of Chemical Engineering, 2019, 27 (11): 2659- 2665. DOI
25	DONG X , GAO Y , LIU C . New normalized Rortex/vortex identification method[J]. Physics of Fluids, 2019, 31 (1): 011701. DOI
26	LIU C Q , WANG Y Q , YANG Y , et al. New omega vortex identification method[J]. Science China, 2016, 59 (8): 684- 711.
27	DONG X , WANG Y , CHEN X , et al. Determination of epsilon for Omega vortex identification method[J]. Journal of Hydrodynamics, 2018, 30 (4): 541- 548. DOI
28	LIU C , GAO Y , TIAN S , et al. Rortex a new vortex vector definition and vorticity tensor and vector decompositions[J]. Physics of Fluids, 2018, 30 (3): 35- 103.
29	YISHENG G , CHAOQUN L . Rortex and comparison with eigenvalue-based vortex identification criteria[J]. Physics of Fluids, 2018, 30 (8): 085107. DOI
30	LIU C . Letter: Galilean invariance of Rortex[J]. Physics of Fluids, 2018, 30 (11): 111701. DOI
31	LIU C , GAO Y S , DONG X R , et al. Third generation of vortex identification methods: Omega and Liutex/Rortex based systems[J]. Journal of Hydrodynamics, 2019, 31 (2): 205- 223. DOI
32	LIU J M , LIU C Q . Modified normalized Rortex/vortex identification method[J]. Fluid Dynamics, 2019, 1905: 03655.
33	黄程蓉, 陈小鹏, 周丹, 等. 黏性溶液中单个气泡的运动形变[J]. 高校化学工程学报, 2018, 32 (5): 1012- 1028.
34	BHAGA D , WEBER M E . Bubbles in viscous liquids: Shapes, wakes and velocities[J]. J Fluid Mech, 1981, 10 (5): 61- 85.
35	蒋炎坤, 褚开星. 基于VOF方法发动机水下排气气泡特性研究[J]. 华中科技大学学报(自然科学版), 2015, 43 (10): 10- 14.

[1]	Li LIU, Shengli NIU, Jinhui ZHU, Yinghong ZUO, Honggang XIE, Peng SHANG. Numerical Simulation of Debris Motion from a Near-space Nuclear Detonation [J]. Chinese Journal of Computational Physics, 2022, 39(5): 521-528.
[2]	Liubin ZHANG, Yanguang SHAN, Zhicheng RONG. Single Bubble Dynamics in Pool Boiling Under Uniform Electric Field: LBM Simulation [J]. Chinese Journal of Computational Physics, 2022, 39(5): 537-548.
[3]	Liyuan WU, Suying ZHANG. Ground State of Bose-Einstein Condensates in a Spin-dependent Optical Lattice [J]. Chinese Journal of Computational Physics, 2022, 39(5): 617-623.
[4]	Xu-lin DU, Lin-song CHENG, Lang-yu NIU, Yu-ming CHEN, Ren-yi CAO, Yong-hong XIE. Numerical Simulation of 3D Discrete Fracture Networks Considering Dynamic Closure of Hydraulic Fractures and Natural Fractures [J]. Chinese Journal of Computational Physics, 2022, 39(4): 453-464.
[5]	Furong GUAN, Chengqian LI, Minyi DENG. Spiral Wave Dynamics of Excited Medium: Effect of Relative Refractory [J]. Chinese Journal of Computational Physics, 2021, 38(6): 749-756.
[6]	Junjie WANG, Jisheng KOU, Jianchao CAI, Yixin PAN, Zhen ZHONG. Tolman Length-based Modified Lucas-Washburn Capillary-driven Model and Numerical Simulation [J]. Chinese Journal of Computational Physics, 2021, 38(5): 521-533.
[7]	Zhankang YANG, Yi NIU. Influence of Temperature, Enclosure and Ventilation on Radon Concentration Distribution in a Blind Roadway [J]. Chinese Journal of Computational Physics, 2021, 38(4): 456-464.
[8]	Qin LOU, Sheng TANG, Haoyuan WANG. Numerical Simulation of Bubble Dynamics in Porous Media with a Lattice Boltzmann Large Density Ratio Model [J]. Chinese Journal of Computational Physics, 2021, 38(3): 289-300.
[9]	HU Shaoliang, XU Xiaowen, ZHENG Yuteng, ZHAO Zhenguo, WANG Weijie, XU Ran, AN Hengbin, MO Zeyao. Algorithms in Linear Solver for Large-scale Time-harmonic Maxwell's Equations in SiP Applications: State-of-the-art and Challenges [J]. Chinese Journal of Computational Physics, 2021, 38(2): 131-145.
[10]	CHEN Hao, CAI Ruming. Shape Optimization of Stratosphere Airship with Propeller [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2020, 37(5): 562-570.
[11]	HU Yu, SUN Tao. Three-dimensional Numerical Simulation of Dynamics Characteristics of Two Rising Bubbles with Lattice Boltzmann Method [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2020, 37(3): 277-283.
[12]	WANG Zimo, LI Ling. Lattice Boltzmann Simulation of Fast Phase Change in Ultrashort Laser Drilling [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2020, 37(3): 299-306.
[13]	SUN Tao, LIU Zhibin, FAN Wei, QIN Haijie. Three-dimensional Numerical Simulation of Vapor Bubble Rising in Superheated Liquid by Lattice Boltzmann Method [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2019, 36(6): 659-664.
[14]	WANG Zhi, ZOU Gaoyu, GONG Jing, BAI Jianfeng, ZHAI Bowen. A Pressure-based Algorithm for Numerical Simulation of One-dimensional Two-phase Flow [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2019, 36(4): 413-420.
[15]	ZHANG Longhui, YOU Changfu. A Fictitious Domain Method Based on Finite Volume Scheme for Particulate Flows [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2019, 36(3): 291-297.