An HLLC Riemann Solver for MHD Tangential Discontinuities

doi:10.19596/j.cnki.1001-246x.8426

Abstract

Abstract:

Compared with those of hydrodynamics, the existence of magnetic field leads to extra characteristic waves for magnetohydrodynamics (MHD), and further leads to an inconsistency of jump condition across the contact discontinuity. Usually, for the magnetic field variables in an HLLC Riemann solver, a single HLL intermediate state is used to replace two HLLC intermediate states to achieve conservation and computational stability, at the cost of insufficient simulation accuracy for tangential discontinuity. In this paper, a previouly developed HLLC solver is specially constructed to deal with MHD tangential discontinuities accurately and satisfy the so-called Toro condition. With numerical tests, such as the time-dependent simulation of one-dimensional shock tube, the tangential discontinuities, and the global MHD simulation of Earth's magnetosphere, we compare numerical results of the modified HLLC solver with those of the standard HLLC and HLLD solvers. It indicates that the modified HLLC solver has better capture accuracy for tangential discontinuities than the previously developed HLLC solver, and has the accuracy of the more time-consuming HLLD solver in some situations.

Key words: magnetohydrodynamics, Riemann solver, HLLC, tangential discontinuities

Xinyue XI, Xiaocheng GUO, Chi WANG. An HLLC Riemann Solver for MHD Tangential Discontinuities[J]. Chinese Journal of Computational Physics, 2022, 39(3): 286-296.

Figures/Tables 6

Fig.1 HLL Riemann fan in x-t plane, showing left state Ul, right state Ur, and middle state U*

Fig.2 HLLC Riemann fan in x-t plane, showing left state Ul, right state Ur, and middle states Ul* and Ur*

Fig.3 Evolution of one-dimensional MHD shocktube

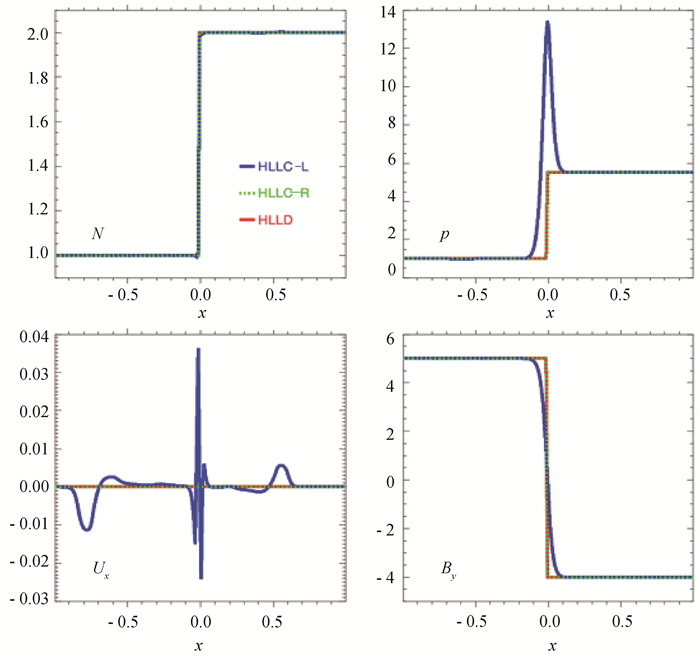
Fig.4 Simulation of a tangential discontinuity for the case of pressure jump across the discontinuity: Spatial profiles of density N, pressure p, velocity component Ux and magnetic field component By

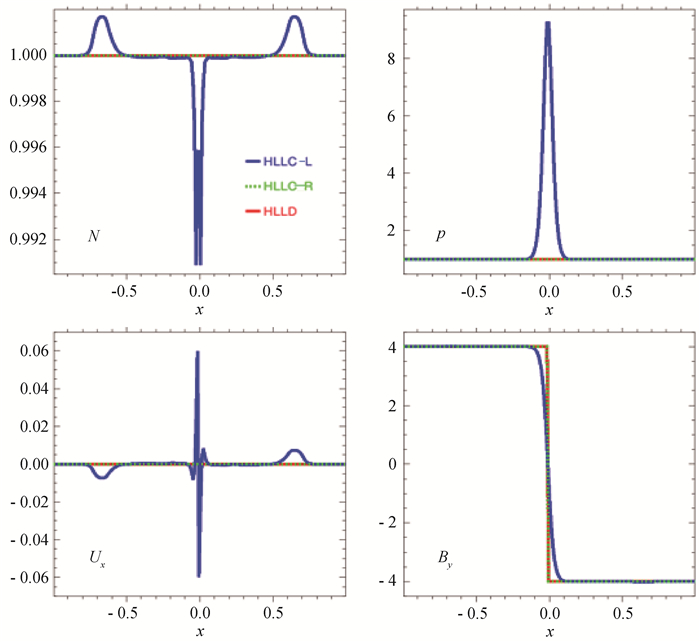
Fig.5 Simulation of a tangential discontinuity for the case of constant pressure across the discontinuity: Spatial profiles of density N, pressure p, velocity component Ux and magnetic field component By

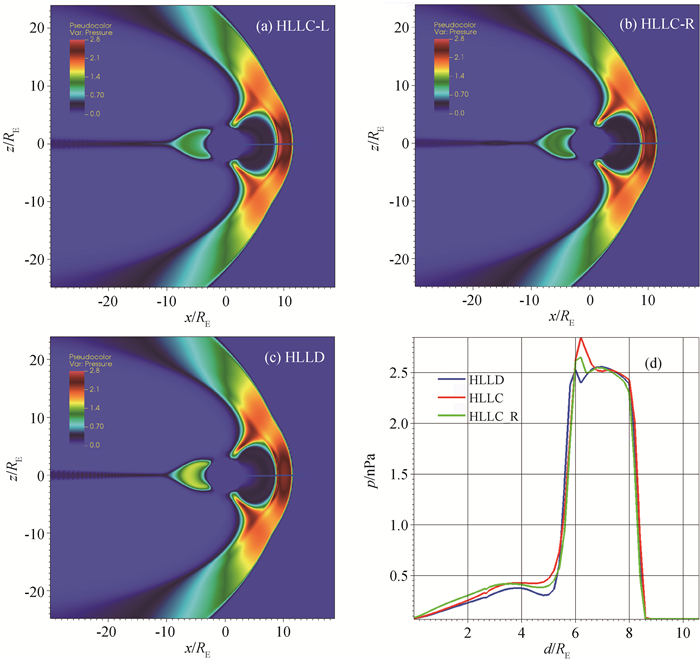
Fig.6 Pressure contours of magnetosphere in equatorial plane (x-z) with three Riemann solvers (a) HLLC-L, (b) HLLC-R, (c) HLLD, and (d) pressure profiles along the Sun-Earth line

References 23

1	傅竹风, 胡友秋. 空间等离子体数值模拟[M]. 合肥: 安徽科学技术出版社, 1995.
2	FENG X . Magnetohydrodynamic modeling of the solar corona and heliosphere[M]. Singapore: Springer, 2020.
3	GODUNOV S K . A difference scheme for numerical solution of discontinuous solution of hydrodynamic equations[J]. Math USSR Sb, 1959, 47, 271- 306.
4	TORO E F. Riemann solvers and numerical methods for fluid dynamics: A practical introduction[M]. 3rd ed. Springer, 2009.
5	ROE P L . Approximate Riemann solvers, parameter vectors, and difference schemes[J]. J Comput Phys, 1981, 43 (2): 357- 372. DOI
6	BRIO M , WU C C . An upwind differencing scheme for the equations of ideal magnetohydrodynamics[J]. J Comput Phys, 1988, 75 (2): 400- 422. DOI
7	BALSARA D S , SPICER D . Maintaining pressure positivity in magnetohydrodynamics simulations[J]. J Comput Phys, 1999, 148 (1): 133- 148. DOI
8	GUO X . An extended HLLC Riemann solver for the magnetohydrodynamics including strong internal magnetic field[J]. J Comput Phys, 2015, 290, 352- 363. DOI
9	GUO X , FLORINSKI V , WANG C . The HLLD Riemann solver based on magnetic field decomposition method for the numerical simulation of magnetohydrodynamics[J]. J Comput Phys, 2016, 327, 543- 552. DOI
10	HARTEN A , LAX P D , VAN LEER B . On upstreaming differencing and Godunov-type schemes for hyperbolic conservation laws[J]. SIAM Rev, 1983, 25 (1): 35- 61. DOI
11	EINFELDT B . On Godunov-type methods for gas dynamics[J]. SIAM J Numer Anal, 1988, 25 (2): 294- 318. DOI
12	EINFELDT B , MUNZ C D , ROE P L , et al. On Godunov-type methods near low densities[J]. J Comput Phys, 1991, 92 (2): 273- 295. DOI
13	TORO E F , SPRUCE M , SPEARES W . Restoration of the contact surface in the HLL-Riemann solver[J]. Shock Waves, 1994, 4 (1): 25- 34. DOI
14	BATTEN P , CLARKE N , LAMBERT C , et al. On the choice of wave speeds for the HLLC Riemann solver[J]. SIAM J Sci Comput, 1997, 18 (6): 1553- 1570. DOI
15	GURSKI K F . An HLLC-type approximate Riemann solver for ideal magnetohydrodynamics[J]. SIAM J Sci Comput, 2004, 25 (6): 2165- 2187. DOI
16	LI S . An HLLC Riemann solver for magnetohydrodynamics[J]. J Comput Phys, 2005, 203 (1): 344- 357. DOI
17	LINDE T . A practical general-purpose two-state HLL Riemann solver for hyperbolic conservation laws[J]. Int J Numer Methods Fluids, 2002, 40 (3/4): 391- 402.
18	MIYOSHI T , KUSANO K . A multi-state HLL approximate Riemann solver for ideal magnetohydrodynamics[J]. J Comput Phys, 2005, 208 (1): 315- 344. DOI
19	FLORINSKI V , GUO X , BALSARA D S , et al. Magnetohydrodynamic modeling of solar system process on geodesic grids[J]. Astrophys J Suppl, 2013, 205 (2): 19. DOI
20	POWELL K G , ROE P L , LINDE T J , et al. A solution-adaptive upwind scheme for ideal magnetohydrodynamics[J]. J Comput Phys, 1999, 154 (2): 284- 309. DOI
21	VAN LEER B . Towards the ultimate conservative difference scheme V: A second-order sequel to Godunov's method[J]. J Comput Phys, 1979, 32 (1): 101- 136. DOI
22	HU Y , GUO X , WANG C . On the ionospheric and reconnection potentials of the Earth: Results from global MHD simulations[J]. J Geophys Res, 2007, 112 (A7): A07215.
23	DE ZEEUW D L , SAZYKIN S , WOLF R A , et al. Coupling of a global MHD code and an inner magnetospheric model: Initial results[J]. J Geophys Res, 2004, 109 (A12): A12219.

[1]	Yaling SHEN, Jianhu FENG, Supei ZHENG, Yarong LI. A High Resolution Entropy Consistent Scheme for Ideal Magnetohydrodynamics Equations with New Slope Limiter [J]. Chinese Journal of Computational Physics, 2022, 39(3): 297-308.
[2]	Liji WANG, Hongping GUO, Zhijun SHEN. Reducing Entropy Production in a Lagrangian Method [J]. Chinese Journal of Computational Physics, 2022, 39(2): 179-190.
[3]	Chuang XUE, Xingdong LI, Wenjun SUN, Wenhua YE, Xianjue PENG. A Multi-material Five-equation-reduced Model and Artificial Compression Method for Interface Capture [J]. Chinese Journal of Computational Physics, 2021, 38(3): 257-268.
[4]	CAO Qiwei, XIAO Delong, YANG Xianjun, WANG Jianguo. Numerical Simulation on Nonlinear Evolution of Magneto-Rayleigh-Taylor Instability [J]. Chinese Journal of Computational Physics, 2021, 38(1): 5-15.
[5]	SUN Chen, LI Xiao, SHEN Zhijun. A Godunov Method with Staggered Lagrangian Discretization Applicable to Isentropic Flows [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2020, 37(5): 529-538.
[6]	LI Xiao, SUN Chen, SHEN Zhijun. Numerical Simulation of One-dimensional Elastic-Perfectly Plastic Flow and Suppression of Wall Heating Phenomenon [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2020, 37(5): 539-550.
[7]	XU Xiao, GAO Zhiming, DAI Zihuan. 3D Lagrangian Methods for Ideal Magnetohydrodynamics on Unstructured Meshes [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2020, 37(4): 403-412.
[8]	LIU Yan, MAO Dekang. An ADRS Approximated Riemann Solver in Compatible Lagrangian Method [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2020, 37(2): 140-152.
[9]	JI Meixuan, YU Xijun, SONG Mingyang. ALE Discontinuous Finite Element Method for One-dimensional Fluid Dynamics Equations with Chemical Reactions [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2020, 37(1): 1-9.
[10]	WU Zongduo, YAN Jin, ZONG Zhi, ZHAO Yong. A Multi-component Mie-Grüneisen Mixture Model Based on HLLC Algorithm [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2020, 37(1): 55-62.
[11]	MAO Jie, XIANG Kai, WANG Yanli, WANG Hao. Numerical Simulation of Magnetohydrodynamic Duct Flow with Sudden Expansion [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2018, 35(5): 597-605.
[12]	REN Jian, SHEN Zhijun, YAN Wei, YUAN Guangwei. A Robust Riemann Solver Without Artificial Intervention [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2018, 35(1): 1-12.
[13]	LIU Jingjing, ZENG Xianyang, NI Guoxi. Euler Numerical Methods for Reactive Flow with General Equation of States in Two Dimensions [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2018, 35(1): 29-38.
[14]	SHANGGUAN Zining, ZHOU Xiuli, SONG Xin, CHEN Qian. Numerical Simulation of Typical Free Surface Flow with SPH-ALE [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2017, 34(6): 641-650.
[15]	DAI Zihuan, WU Jiming, DING Ning. Artificial Viscosity Splitting Strategy in Lagrangian Algorithm for Two-dimensional Three-temperature Radiation Magnetohydrodynamics [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2015, 32(4): 379-385.