Low-dose X-ray CT Image Reconstruction Method Based on Adaptive Total Variation

doi:10.19596/j.cnki.1001-246x.8639

Abstract

Abstract:

The radiation dose of X-ray CT is closely related to the abnormal metabolism of the living body and the induction of diseases such as cancer. At this stage, how to reduce the radiation dose of CT scans and ensure the quality of image reconstruction is a huge challenge for CT image reconstruction technology. The traditional total variation regularization method is not sensitive to the direction of the image. This paper introduces directional gradient information and establishes an adaptive directional total variation sparse angle CT image reconstruction model, which aims to better preserve image edges and improve image reconstruction quality. To adaptively estimate the direction information of the image gradient, based on the geometric moment of the image, an ellipse steerable kernel is used to estimate the main direction of the image edge block. Simulation and actual data results show that the proposed algorithm has good performance in image detail preservation, artifact reduction, and noise suppression.

Key words: low-dose, X-ray CT, sparse-view reconstruction, gradient direction, total variation

Zhaoyan QU, Ximing YAN, Xiaojing XI. Low-dose X-ray CT Image Reconstruction Method Based on Adaptive Total Variation[J]. Chinese Journal of Computational Physics, 2023, 40(5): 614-621.

Figures/Tables 9

Fig.1 Reconstruction results of TV algorithm (a) traditional TV; (b) TV gradient directions of 45°; (c) TV gradient directions of 100°; (d) TV gradient directions of 130°; (f) TV gradient directions of 160°; (f) adaptive TV

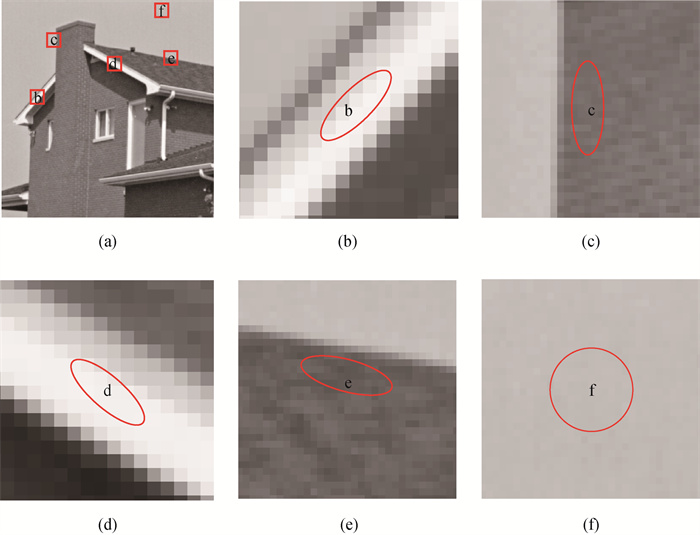
Fig.2 Adaptive gradient difference direction

Fig.3 Estimation of the direction of the house image block (a) house image; (b) left diagonal edge; (c) vertical edge; (d) right diagonal edge; (e) horizontal edge; (f) smooth area

Fig.4 Graph of directional gradient operator

Fig.5 Comparison of medical reconstruction results

Fig.6 PSNR of four different algorithms with the number of sparse views of (a) 30; (b) 60; (c) 90

Fig.7 Phantom, grayscale range [0, 0.035]

Fig.8 Image profifiles marked by green lines in Fig. 7 with the number of sparse views of (a) 30; (b) 60; (c) 90

Fig.9 Image profifiles marked by red lines in Fig. 7 with the number of sparse views of (a) 30; (b) 60; (c) 90

References 22

1	BRENNER D . Computed tomography-an increasing source of radiation exposure[J]. The New England Journal of Medicine, 2007, 357 (22): 2277- 2284. DOI
2	MCCOLLOUGH C , BRUESEWITZ M , KOFLER J . CT dose reduction and dose management tools: Overview of available options[J]. Radiographics, 2006, 26 (2): 503- 512. DOI
3	PARCERO E , FLORES L , et al. Impact of view reduction in CT on radiation dose for patients[J]. Radiation Physics and Chemistry, 2017, 137 (5): 173- 175.
4	LI J , PENG H , ZHANG G , et al. Theoretical study of X-ray laser holography[J]. Chinese Journal of Computational Physics, 1997, (Z1): 40- 42.
5	LIU Z , M Y . Monte Carlo simulation of reverse nuclear explosion equivalent from X-ray imaging[J]. Chinese Journal of Computational Physics, 2010, 27 (1): 15- 22.
6	BULS N , VAN G G , VAN C T , et al. Contrast agent and radiation dose reduction in abdominal CT by a combination of low tube voltage and advanced image reconstruction algorithms[J]. European Radiology, 2014, 25 (4): 1023- 1031.
7	SIDKY E Y , KAO C M , PAN X . Accurate image reconstruction from few-views and limited-angle data in divergent-beam CT[J]. Journal of X-ray Science and Technology, 2009, 14 (2): 119- 139.
8	LOUIS A K , RIEDER A . Incomplete data problems in X-ray computerized tomography[J]. Numerische Mathematik, 1998, 56 (4): 371- 383.
9	YAN X , YANG J , YANG Y . Fast filtered back projection algorithm in image reconstruction of Compton camera[J]. Chinese Journal of Computational Physics, 2020, 37 (2): 153- 162.
10	GORDON R , BENDER R , HERMAN G T . Algebraic reconstruction techniques (ART) for three-dimensional electron microscopy and X-ray photography[J]. Theoretical Biology, 1970, 29 (3): 471- 481. DOI
11	ANDERS A H . Algebraic reconstruction in CT from limited views[J]. IEEE Transactions on Medical Imaging, 1989, 8 (1): 50- 55. DOI
12	CANDES E J , ROMBERG J , TAO T . Robust uncertainty principles: Exact signal reconstruction from highly incomplete frequency information[J]. IEEE Transactions on Information Theory, 2006, 52 (2): 489- 509. DOI
13	TIKHONOV A N , ARSENIN V Y . Solutions of ill-posed problems[J]. Mathematics of Computation, 1977, 32 (144): 491- 491.
14	RUDIN L I , OSHER S , FATATEMI E . Nonlinear total variation based noise removal algorithms[J]. Physica D, 1992, 60 (4): 259- 268.
15	SIDKY E Y , PAN X . Image reconstruction in circular cone-beam computed tomography by constrained total-variation minimization[J]. Physics in Medicine and Biology, 2013, 53 (17): 4777- 4807.
16	BREDIES K , KUNISCH K , POCK T . Total generalized variation[J]. Siam Journal on Imagingences, 2010, 3 (3): 492- 526. DOI
17	TIAN Z , JIA X , YUAN K , et al. Low-dose CT reconstruction via edge-preserving total variation regularization[J]. Physics in Medicine and Biology, 2011, 56 (18): 5949- 5967. DOI
18	KIM H , CHEN J , WANG A , et al. Non-local total-variation (NLTV) minimization combined with reweighted L1-norm for compressed sensing CT reconstruction[J]. Physics in Medicine and Biology, 2016, 61 (18): 6878- 6891. DOI
19	CHEN Z , JIN X , LI L , et al. A limited-angle CT reconstruction method based on anisotropic TV minimization[J]. Physics in Medicine and Biology, 2013, 58 (7): 2119- 2141. DOI
20	LIU Y , MA J , FAN Y , et al. Adaptive-weighted total variation minimization for sparse data toward low-dose X-ray computed tomography image reconstruction[J]. Physics in Medicine & Biology, 2012, 57 (23): 7923- 7956.
21	GUO Y , ZENG L , WANG C , et al. Image reconstruction model for the exterior problem of computed tomography based on weighted directional total variation[J]. Applied Mathematical Modelling, 2017, 52 (12): 358- 377.
22	TEAGUE M R . Image analysis via the general theory of moments[J]. JOSA, 1980, 70 (8): 920- 930. DOI

[1]	GE Quanwen, LIN Zhong, WANG Ruili. Formulation of Edge-centered Artificial Viscosity Unstructured Mesh [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 2008, 25(1): 22-28.
[2]	Jin Baoxia. AN ESSENTIALLY CONSERVATIVE SCHEME FOR 2D HYPERBOLIC CONSERVATION LAWS [J]. CHINESE JOURNAL OF COMPUTATIONAL PHYSICS, 1994, 11(3): 337-345.