Chinese Journal of Computational Physics ›› 2024, Vol. 41 ›› Issue (5): 619-629.DOI: 10.19596/j.cnki.1001-246x.8793
• Research Reports • Previous Articles Next Articles
Xingkang LIU1( ), Xingding CHEN1, Yunlong YU2,*(
), Xingding CHEN1, Yunlong YU2,*( )
)
Received:2023-07-03
Online:2024-09-25
Published:2024-09-14
Contact:
Yunlong YU
CLC Number:
Xingkang LIU, Xingding CHEN, Yunlong YU. Deflated Preconditioned Conjugate Gradient Solvers for Linear Elastic Crack Problems[J]. Chinese Journal of Computational Physics, 2024, 41(5): 619-629.
Add to citation manager EndNote|Ris|BibTeX
URL: http://www.cjcp.org.cn/EN/10.19596/j.cnki.1001-246x.8793
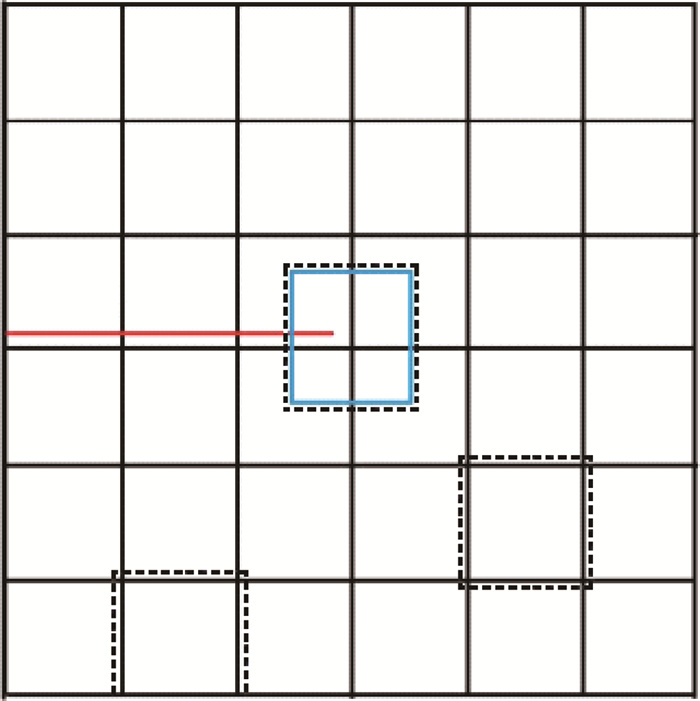
Fig.2 Schematic of the "crack tip" domain decomposition (The blue subdomain denotes the "crack tip" subdomain, and the black subdomains denote "regular" subdomains.)
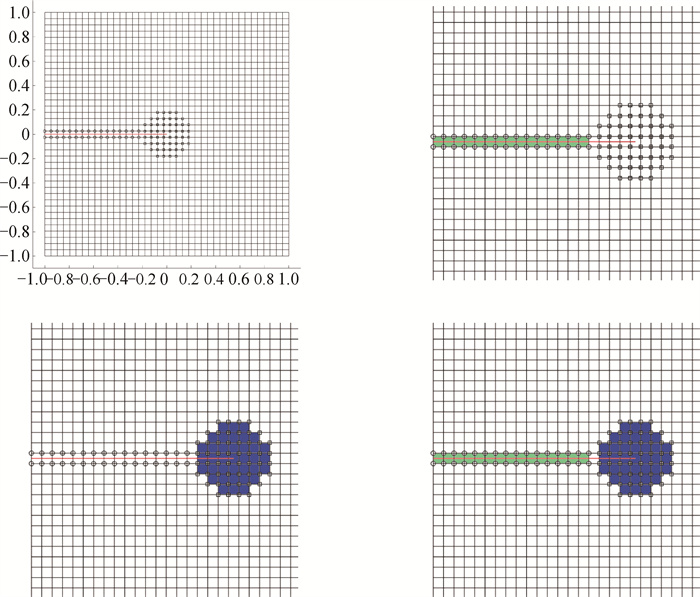
Fig.4 Schematic of deflation nodes selections in geometric XFEM (a) the mesh is dissected into 39×39; (b) only Heaviside enhancement nodes are selected; (c) only crack-tip enhancement nodes are selected; (d) all enhancement nodes are selected ((b)-(d) are the schematic representations of the different point selection strategies after local magnification.)
| 网格剖分 | CGJac | ADCGH | ADCGtip | ADCGHtip |
| 19×19 | 28 | 21 | 27 | 22 |
| 39×39 | 41 | 32 | 39 | 31 |
| 59×59 | 50 | 38 | 48 | 38 |
| 79×79 | 58 | 44 | 55 | 43 |
| 99×99 | 64 | 49 | 61 | 48 |
| 119×119 | 69 | 53 | 66 | 52 |
| 139×139 | 74 | 57 | 71 | 55 |
Table 1 The number of iterations for different selection of deflated nodes
| 网格剖分 | CGJac | ADCGH | ADCGtip | ADCGHtip |
| 19×19 | 28 | 21 | 27 | 22 |
| 39×39 | 41 | 32 | 39 | 31 |
| 59×59 | 50 | 38 | 48 | 38 |
| 79×79 | 58 | 44 | 55 | 43 |
| 99×99 | 64 | 49 | 61 | 48 |
| 119×119 | 69 | 53 | 66 | 52 |
| 139×139 | 74 | 57 | 71 | 55 |
| 网格剖分 | ADCGH | ADCGtip | ADCGHtip |
| 19×19 | 16 | 12 | 28 |
| 39×39 | 32 | 52 | 84 |
| 59×59 | 48 | 112 | 160 |
| 79×79 | 64 | 192 | 256 |
| 99×99 | 80 | 308 | 388 |
| 119×119 | 96 | 448 | 544 |
| 139×139 | 112 | 608 | 720 |
Table 2 The number of nodes included in different nodes selection strategies
| 网格剖分 | ADCGH | ADCGtip | ADCGHtip |
| 19×19 | 16 | 12 | 28 |
| 39×39 | 32 | 52 | 84 |
| 59×59 | 48 | 112 | 160 |
| 79×79 | 64 | 192 | 256 |
| 99×99 | 80 | 308 | 388 |
| 119×119 | 96 | 448 | 544 |
| 139×139 | 112 | 608 | 720 |
| 网格剖分 | CondNPre | CondJac | CondAS | CondRAS |
| 19×19 | 2.25×106 | 2.34×105 | 8.24×102 | 6.75×102 |
| 39×39 | 1.62×109 | 6.81×107 | 1.04×105 | 1.02×105 |
| 59×59 | 3.05×1010 | 1.44×109 | 1.23×106 | 1.23×106 |
| 79×79 | 1.86×1011 | 1.08×1010 | 7.77×106 | 7.76×106 |
| 99×99 | 1.43×1012 | 1.35×1011 | 5.08×107 | 5.07×107 |
Table 3 The condition number of M-1A after different preconditioning (δ=2)
| 网格剖分 | CondNPre | CondJac | CondAS | CondRAS |
| 19×19 | 2.25×106 | 2.34×105 | 8.24×102 | 6.75×102 |
| 39×39 | 1.62×109 | 6.81×107 | 1.04×105 | 1.02×105 |
| 59×59 | 3.05×1010 | 1.44×109 | 1.23×106 | 1.23×106 |
| 79×79 | 1.86×1011 | 1.08×1010 | 7.77×106 | 7.76×106 |
| 99×99 | 1.43×1012 | 1.35×1011 | 5.08×107 | 5.07×107 |
| 网格剖分 | ADCGJac | ADCGEAS | ADCGERAS | ADCGAS | ADCGRAS |
| 19×19 | 21 | 3 | 3 | 3 | 3 |
| 39×39 | 32 | 5 | 5 | 5 | 5 |
| 59×59 | 38 | 7 | 7 | 9 | 10 |
| 79×79 | 44 | 9 | 10 | 11 | 12 |
| 99×99 | 49 | 13 | 13 | 16 | 18 |
| 119×119 | 53 | 13 | 16 | 20 | 22 |
| 139×139 | 57 | 15 | 17 | 23 | 25 |
Table 4 The number of iterations of ADCG method using different preconditioners M-1 (Overlap δ=2 and E denotes exact solution in the regular domain.)
| 网格剖分 | ADCGJac | ADCGEAS | ADCGERAS | ADCGAS | ADCGRAS |
| 19×19 | 21 | 3 | 3 | 3 | 3 |
| 39×39 | 32 | 5 | 5 | 5 | 5 |
| 59×59 | 38 | 7 | 7 | 9 | 10 |
| 79×79 | 44 | 9 | 10 | 11 | 12 |
| 99×99 | 49 | 13 | 13 | 16 | 18 |
| 119×119 | 53 | 13 | 16 | 20 | 22 |
| 139×139 | 57 | 15 | 17 | 23 | 25 |
| 网格剖分 | δ=1 | δ=2 | δ=3 | |||||
| ADCGEAS | ADCGERAS | ADCGEAS | ADCGERAS | ADCGEAS | ADCGERAS | |||
| 19×19 | 4 | 3 | 3 | 3 | 3 | 3 | ||
| 39×39 | 5 | 5 | 5 | 5 | 5 | 5 | ||
| 59×59 | 9 | 7 | 7 | 7 | 7 | 7 | ||
| 79×79 | 9 | 14 | 9 | 10 | 9 | 10 | ||
| 99×99 | 15 | 16 | 13 | 13 | 11 | 13 | ||
| 119×119 | 17 | 18 | 13 | 16 | 13 | 14 | ||
| 139×139 | 19 | 19 | 15 | 17 | 13 | 15 | ||
Table 5 The number of iterations of ADCG method with different overlap δ
| 网格剖分 | δ=1 | δ=2 | δ=3 | |||||
| ADCGEAS | ADCGERAS | ADCGEAS | ADCGERAS | ADCGEAS | ADCGERAS | |||
| 19×19 | 4 | 3 | 3 | 3 | 3 | 3 | ||
| 39×39 | 5 | 5 | 5 | 5 | 5 | 5 | ||
| 59×59 | 9 | 7 | 7 | 7 | 7 | 7 | ||
| 79×79 | 9 | 14 | 9 | 10 | 9 | 10 | ||
| 99×99 | 15 | 16 | 13 | 13 | 11 | 13 | ||
| 119×119 | 17 | 18 | 13 | 16 | 13 | 14 | ||
| 139×139 | 19 | 19 | 15 | 17 | 13 | 15 | ||
| 网格剖分 | 5×5 | 7×7 | 9×9 | |||||
| CGERAS | CGRAS | CGERAS | CGRAS | CGERAS | CGRAS | |||
| 39×39 | 21 | 23 | 28 | 29 | 27 | 31 | ||
| 59×59 | 25 | 27 | 30 | 32 | 35 | 42 | ||
| 79×79 | 29 | 37 | 33 | 48 | 39 | 60 | ||
| 99×99 | 32 | 42 | 37 | 56 | 43 | 86 | ||
| 119×119 | 36 | 43 | 39 | 57 | 48 | 93 | ||
| 139×139 | 38 | 60 | 43 | 68 | 48 | 101 | ||
Table 6 The number of iterations for M RAS-1 preconditioned CG method with multi-subdomains
| 网格剖分 | 5×5 | 7×7 | 9×9 | |||||
| CGERAS | CGRAS | CGERAS | CGRAS | CGERAS | CGRAS | |||
| 39×39 | 21 | 23 | 28 | 29 | 27 | 31 | ||
| 59×59 | 25 | 27 | 30 | 32 | 35 | 42 | ||
| 79×79 | 29 | 37 | 33 | 48 | 39 | 60 | ||
| 99×99 | 32 | 42 | 37 | 56 | 43 | 86 | ||
| 119×119 | 36 | 43 | 39 | 57 | 48 | 93 | ||
| 139×139 | 38 | 60 | 43 | 68 | 48 | 101 | ||
| 网格剖分 | ADCGJac | 5×5 | 7×7 | 9×9 | |||||
| ADCGEAS | ADCGERAS | ADCGEAS | ADCGERAS | ADCGEAS | ADCGERAS | ||||
| 39×39 | 32 | 11 | 11 | 15 | 14 | 16 | 15 | ||
| 59×59 | 38 | 14 | 13 | 16 | 19 | 18 | 20 | ||
| 79×79 | 44 | 13 | 15 | 16 | 16 | 20 | 20 | ||
| 99×99 | 49 | 14 | 14 | 17 | 17 | 25 | 25 | ||
| 119×119 | 53 | 15 | 15 | 18 | 18 | 27 | 27 | ||
| 139×139 | 57 | 20 | 20 | 19 | 19 | 27 | 27 | ||
Table 7 The number of iterations for different preconditioners with multi-subdomains
| 网格剖分 | ADCGJac | 5×5 | 7×7 | 9×9 | |||||
| ADCGEAS | ADCGERAS | ADCGEAS | ADCGERAS | ADCGEAS | ADCGERAS | ||||
| 39×39 | 32 | 11 | 11 | 15 | 14 | 16 | 15 | ||
| 59×59 | 38 | 14 | 13 | 16 | 19 | 18 | 20 | ||
| 79×79 | 44 | 13 | 15 | 16 | 16 | 20 | 20 | ||
| 99×99 | 49 | 14 | 14 | 17 | 17 | 25 | 25 | ||
| 119×119 | 53 | 15 | 15 | 18 | 18 | 27 | 27 | ||
| 139×139 | 57 | 20 | 20 | 19 | 19 | 27 | 27 | ||
| 网格剖分 | ADCGJac | 5×5 | 7×7 | 9×9 | |||||
| ADCGAS | ADCGRAS | ADCGAS | ADCGRAS | ADCGAS | ADCGRAS | ||||
| 39×39 | 32 | 11 | 11 | 14 | 14 | 16 | 16 | ||
| 59×59 | 38 | 12 | 14 | 17 | 17 | 18 | 21 | ||
| 79×79 | 44 | 14 | 13 | 16 | 16 | 20 | 20 | ||
| 99×99 | 49 | 16 | 14 | 17 | 17 | 25 | 25 | ||
| 119×119 | 53 | 21 | 15 | 21 | 21 | 29 | 26 | ||
| 139×139 | 57 | 22 | 20 | 22 | 22 | 28 | 27 | ||
Table 8 The number of iterations for different preconditioners with multi-subdomains (Inexact solutions using ILU are used in the regular domain.)
| 网格剖分 | ADCGJac | 5×5 | 7×7 | 9×9 | |||||
| ADCGAS | ADCGRAS | ADCGAS | ADCGRAS | ADCGAS | ADCGRAS | ||||
| 39×39 | 32 | 11 | 11 | 14 | 14 | 16 | 16 | ||
| 59×59 | 38 | 12 | 14 | 17 | 17 | 18 | 21 | ||
| 79×79 | 44 | 14 | 13 | 16 | 16 | 20 | 20 | ||
| 99×99 | 49 | 16 | 14 | 17 | 17 | 25 | 25 | ||
| 119×119 | 53 | 21 | 15 | 21 | 21 | 29 | 26 | ||
| 139×139 | 57 | 22 | 20 | 22 | 22 | 28 | 27 | ||
| 1 | 徐小文, 莫则尧, 胡少亮, 等. 特征修正并行预条件算法框架[J]. 计算物理, 2024, 41 (1): 64- 74. |
| 2 | 杜旭林, 程林松, 牛烺昱, 等. 考虑水力压裂缝和天然裂缝动态闭合的三维离散缝网数值模拟[J]. 计算物理, 2022, 39 (4): 453- 464. |
| 3 |
COLOMBO D , GIGLIO M . A methodology for automatic crack propagation modelling in planar and shell FE models[J]. Engineering Fracture Mechanics, 2006, 73 (4): 490- 504.
DOI |
| 4 | 余天堂. 扩展有限单元法——理论、应用及程序[M]. 北京: 科学出版社, 2014: 1- 12. |
| 5 |
BELYTSCHKO T , BLACK T . Elastic crack growth in finite elements with minimal remeshing[J]. International Journal for Numerical Methods in Engineering, 1999, 45 (5): 601- 620.
DOI |
| 6 |
MOËS N , DOLBOW J , BELYTSCHKO T . A finite element method for crack growth without remeshing[J]. International Journal for Numerical Methods in Engineering, 1999, 46 (1): 131- 150.
DOI |
| 7 |
DAUX C , MOËS N , DOLBOW J , et al. Arbitrary branched and intersecting cracks with the extended finite element method[J]. International Journal for Numerical Methods in Engineering, 2000, 48 (12): 1741- 1760.
DOI |
| 8 | MELENK J M , BABUŠKA I . The partition of unity finite element method: Basic theory and applications[J]. Computer Methods in Applied Mechanics and Engineering, 1996, 139 (1/4): 289- 314. |
| 9 |
BABUŠKA I , BANERJEE U . Stable generalized finite element method (SGFEM)[J]. Computer Methods in Applied Mechanics and Engineering, 2012, 201/204, 91- 111.
DOI |
| 10 |
GUPTA V , DUARTE C A , BABUŠKA I , et al. A stable and optimally convergent generalized FEM (SGFEM) for linear elastic fracture mechanics[J]. Computer Methods in Applied Mechanics and Engineering, 2013, 266, 23- 39.
DOI |
| 11 |
ZHANG Qinghui , BABUŠKA I , BANERJEE U . Robustness in stable generalized finite element methods (SGFEM) applied to Poisson problems with crack singularities[J]. Computer Methods in Applied Mechanics and Engineering, 2016, 311, 476- 502.
DOI |
| 12 |
TIAN Rong , WEN Longfei , WANG Lixiang . Three-dimensional improved XFEM (IXFEM) for static crack problems[J]. Computer Methods in Applied Mechanics and Engineering, 2019, 343, 339- 367.
DOI |
| 13 |
MENK A , BORDAS S P A . A robust preconditioning technique for the extended finite element method[J]. International Journal for Numerical Methods in Engineering, 2011, 85 (13): 1609- 1632.
DOI |
| 14 |
BERGER-VERGIAT L , WAISMAN H , HIRIYUR B , et al. Inexact Schwarz-algebraic multigrid preconditioners for crack problems modeled by extended finite element methods[J]. International Journal for Numerical Methods in Engineering, 2012, 90 (3): 311- 328.
DOI |
| 15 |
CHEN Xingding , CAI Xiaochuan . Effective two-level domain decomposition preconditioners for elastic crack problems modeled by extended finite element method[J]. Communications in Computational Physics, 2020, 28 (4): 1561- 1584.
DOI |
| 16 |
AGATHOS K , DODWELL T , CHATZI E , et al. An adapted deflated conjugate gradient solver for robust extended/generalised finite element solutions of large scale, 3D crack propagation problems[J]. Computer Methods in Applied Mechanics and Engineering, 2022, 395, 114937.
DOI |
| 17 |
SAAD Y , YEUNG M , ERHEL J , et al. A deflated version of the conjugate gradient algorithm[J]. SIAM Journal on Scientific Computing, 2000, 21 (5): 1909- 1926.
DOI |
| 18 |
NICOLAIDES R A . Deflation of conjugate gradients with applications to boundary value problems[J]. SIAM Journal on Numerical Analysis, 1987, 24 (2): 355- 365.
DOI |
| 19 | TANG J M, NABBEN R, VUIK C, et al. Theoretical and numerical comparison of various projection methods derived from deflation, domain decomposition and multigrid methods: REPORT 07-04[R]. Delft: Delft University of Technology, 2007. |
| 20 |
PARKS M L , DE STURLER E , MACKEY G , et al. Recycling Krylov subspaces for sequences of linear systems[J]. SIAM Journal on Scientific Computing, 2006, 28 (5): 1651- 1674.
DOI |
| 21 |
DIAZ CORTES G B , VUIK C , JANSEN J D . On POD-based deflation vectors for DPCG applied to porous media problems[J]. Journal of Computational and Applied Mathematics, 2018, 330, 193- 213.
DOI |
| 22 | 李开泰, 黄艾香, 黄庆怀. 有限元方法及其应用[M]. 北京: 科学出版社, 2006: 54- 64. |
| 23 |
AUBRY R , MUT F , DEY S , et al. Deflated preconditioned conjugate gradient solvers for linear elasticity[J]. International Journal for Numerical Methods in Engineering, 2011, 88 (11): 1112- 1127.
DOI |
| 24 |
CAI Xiaochuan , SARKIS M . A restricted additive schwarz preconditioner for general sparse linear systems[J]. SIAM Journal on Scientific Computing, 1999, 21 (2): 792- 797.
DOI |
| 25 | 范鹤潇, 陈星玎. 一类求解含静态裂缝线弹性问题的预条件扩展有限元方法[J]. 计算物理, 2024, 41 (2): 151- 160. |
| [1] | Hexiao FAN, Xingding CHEN. A Class of Preconditioners for Static Elastic Crack Problems Modeled by Extended Finite Element Method [J]. Chinese Journal of Computational Physics, 2024, 41(2): 151-160. |
| Viewed | ||||||
|
Full text |
|
|||||
|
Abstract |
|
|||||
Copyright © Chinese Journal of Computational Physics
E-mail: jswl@iapcm.ac.cn
Supported by Beijing Magtech Co., Ltd.
