基于维格纳方程的电子的古斯-汉欣位移

doi:10.19596/j.cnki.1001-246x.8310

摘要/Abstract

摘要：

通过求解电子的维格纳方程研究二维电子气中电子的输运性质。我们发现电子在倾斜入射到势垒界面并反射时, 出现与光波类似的古斯-汉欣位移。通过维格纳方程可以得到电子的瞬态演化, 不仅可以计算古斯-汉欣位移还能研究电子在势垒内部的运动轨迹以及出现稳定古斯-汉欣位移的时间。与稳定相位法得到的古斯-汉欣位移对比发现, 考虑古斯-汉欣位移的界面反射较几何光学反射在时间上有一定迟缓, 这种迟缓与入射角无关, 但会随着势垒宽度的增加而增加; 电子的古斯-汉欣位移与势垒厚度无关, 随着入射角或入射能量的增大而增大。基于此, 我们提出一种电子分束器模型, 向输入端注入初始动能不同的高斯波包, 当电子能量低于0.01 eV时, 约85%的电子运动至第二输出端; 而当电子能量高于0.07 eV时, 约85%的电子运动至第一输出端。

关键词: 古斯-汉欣位移, 维格纳方程, 电子分束器, 平面纳米器件

Abstract:

We studied transport properties of electrons in two-dimensional electron gas with Wigner equation. It was found that as electrons enter the barrier interface obliquely and are reflected, Goos-Hanchen shift similar to light waves presents. With Wigner equation we get transient evolution of electrons, with which we can calculate Goos-Hanchen shift and study trajectory of electrons inside the potential barrier as well. Compared with Goos-Hanchen shift obtained with the stable phase method, it is found that the interface reflection considering Goos-Hanchen shift has a certain retardation in time compared with geometric optical reflection, which is independent of incident angle, but increases with the increase of width. However, Goos-Hanchen shift of electrons is independent of barrier width and increases with the incident angle or incident energy. According to this, we propose an electron beam splitter model in which Gaussian wave packets with different initial kinetic energies inject into the input. As electron energy is below 0. 01 eV, about 85% of the electrons move to the second output, while as electron energy is above 0. 07 eV, about 85% of the electrons move to the first output.

Key words: Goos-Hanchen shift, Wigner formalism, electron beam splitter, planar nanometer device

中图分类号:

O463

程受广, 尹云倩, 钟菊莲, 许坤远. 基于维格纳方程的电子的古斯-汉欣位移[J]. 计算物理, 2021, 38(4): 431-440.

Shouguang CHENG, Yunqian YIN, Julian ZHONG, Kunyuan XU. Goos-Hanchen Shift of Electrons Based on Wigner Equation[J]. Chinese Journal of Computational Physics, 2021, 38(4): 431-440.

图/表 12

图1 基于符号粒子的维格纳蒙特卡罗方法流程图

Fig.1 Flowchart of the Wigner Monte Carlo methodbased on signed particles

表1 模拟参数

Table 1 Simulation parameters

L_x/nm	L_y/nm	L_c/nm	Δk/nm^-1	Δl/fs	Δx/nm	Δy/nm	k_x0/nm^-1	σ/nm	势垒宽度a/nm	势垒高度/eV
150	150	100	π/L_c	1.0	1.0	1.5	0.25	10	10	0.05

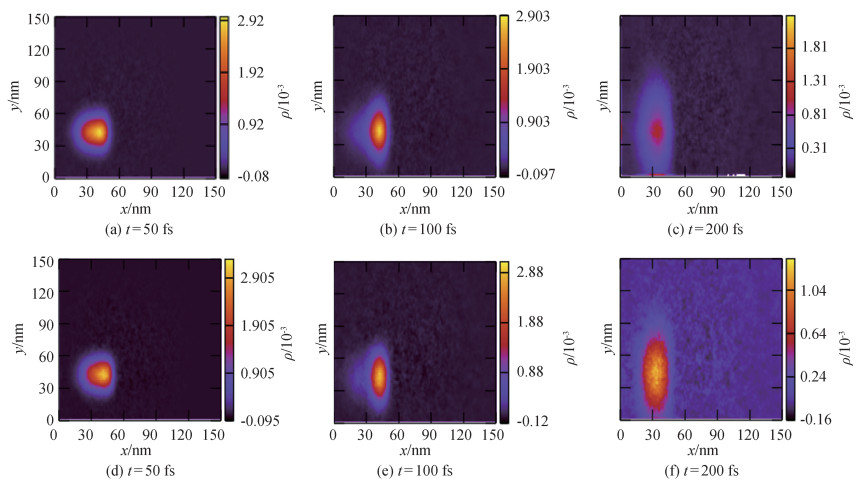
图2 优化再分配方案对比(a), (b), (c)为原程序密度分布；(d), (e), (f)为优化后密度分布

Fig.2 Comparison of optimizing redistribution scheme (a), (b), (c) are density distributions by the original program; (d), (e), (f) represent density distributions after optimization

表2 方势垒演化的线程-加速比

Table 2 Thread-acceleration ratios of thesquare barrier evolution

线程数	计算时间/s	加速比
1	94 443	1
2	54 627	1.73
3	36 064	2.62
4	30 710	3.08
5	28 684	3.29
6	23 071	4.09
7	21 187	4.45
8	19 285	4.9

图3 光在界面处的全反射及古斯-汉欣位移

Fig.3 Total reflection of light at interface andGoos-Hanchen shift

图4 波包的中心轨迹与几何光学反射

Fig.4 Total reflection of light and Goos-Hanchen shift

图5 势垒宽度对古斯-汉欣位移的影响

Fig.5 Influence of barrier width on Goos-Hanchen shift

图6 入射角度对古斯-汉欣位移的影响

Fig.6 Influence of incident angle on Goos-Hanchen shift

图7 入射能量对古斯-汉欣位移的影响

Fig.7 Influence of incident energy on Goos-Hanchen shift

图8 器件结构

Fig.8 Device structure

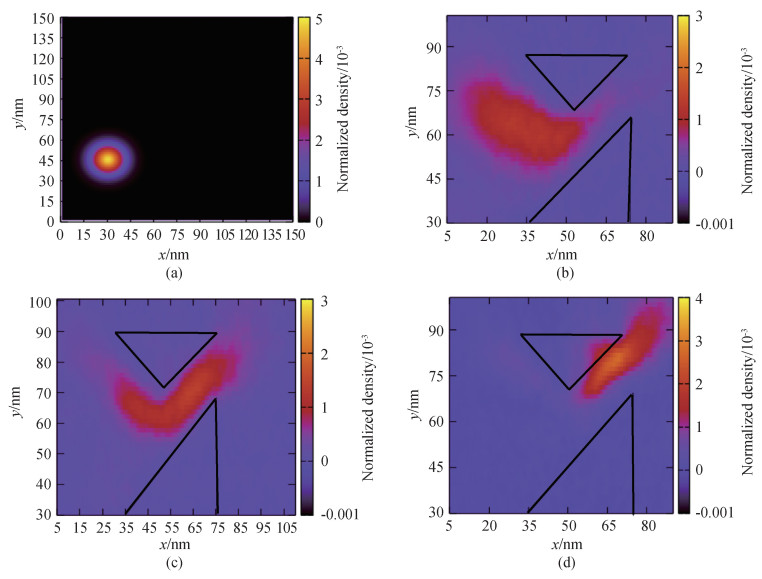
图9 不同入射能量的波包在势垒界面的运动(a)初始时刻波包位置；(b)入射动能E=0.01 eV波包在t=270 fs时的位置；(c)入射动能E=0.028 eV波包在t=180 fs时的位置；(d)入射动能E=0.07 eV波包在t=140 fs时的位置

Fig.9 Movement of wave packets with different incident energy at the barrier interface (a) Initial position of the wave packet; (b) Position of a incident kinetic energy E=0.01 eV wave packet at t=270 fs; (c) Position of a incident kinetic energy E=0.028 eV wave packet at t=180 fs; (d) Position of a incident kinetic energy E=0.0 7 eV wave packet at t=140 fs

图10 不同入射动能的波包到达两个输出端的比率

Fig.10 The ratio of wave packets to two output terminalswith different incident kinetic energy

参考文献 28

1	GOOS F, HÄNCHEN H. Ein neuer und fundamentaler versuch zur total reflexion[J]. Annalen der Physik, 1947, 436 (7-8): 333- 346. DOI
2	GOOS F, HÄNCHEN H. Neumessung des Strahlversetzung seffektes bei total reflexion[J]. Annalen der Physik, 1949, 440 (3-5): 251- 252. DOI
3	ARTMANN K. Berechnung der Seitenversetzung des total reflektierten Strahles[J]. Annalen der Physik, 1948, 437 (1-2): 87- 102. DOI
4	RENARD R H. Total reflection: A new evaluation of the Goos-Hänchen shift[J]. Journal of the Optical Society of America, 1964, 54 (10): 1190- 1197. DOI
5	YU Z M, LIU Y, YANG S A. Anomalous spatial shifts in interface electronic scattering[J]. Frontiers of Physics, 2019, 14 (3): 33402. DOI
6	BLIOKH K Y, AIELLO A. Goos-Hänchen and Imbert-Fedorov beam shifts: An overview[J]. Journal of Optics, 2013, 15 (1): 014001. DOI
7	SINITSYN N A, NIU Q, SINOVA J, et al. Disorder effects in the anomalous Hall effect induced by Berry curvature[J]. Physical Review B, 2005, 72 (4): 045346. DOI
8	BALLICCHIA M, WEINBUB J, NEDJALKOV . Electron evolution around a repulsive dopant in a quantum wire: Coherence effects[J]. Nanoscale, 2018, 10 (48): 23037- 23049. DOI
9	SELLIER J M, KAPANOVA K G. A study of entangled systems in the many-body signed particle formulation of quantum mechanics[J]. International Journal of Quantum Chemistry, 2017, 117 (23): e25447. DOI
10	WIGNER E P. On the quantum correction for thermodynamic equilibrium[J]. Physical Review, 1932, 40, 749. DOI
11	SELLIER J M. A signed particle formulation of non-relativistic quantum mechanics[J]. Journal of Computational Physics, 2015, 297, 254- 265. DOI
12	SELLIER J M, NEDJALKOV M, DIMOV I, et al. A benchmark study of the Wigner Monte Carlo method[J]. Monte Carlo Methods and Applications, 2014, 20 (1): 43- 51.
13	WEINBUB J, FERRY D K. Recent advances in Wigner function approaches[J]. Applied Physics Reviews, 2018, 5 (4): 041104. DOI
14	KIM K Y, LEE B. On the high order numerical calculation schemes for the Wigner transport equation[J]. Solid-State Electronics, 1999, 43 (12): 2243- 2245. DOI
15	KIM K Y, KIM S, TANG T. Accuracy balancing for the finite-difference-based solution of the discrete Wigner transport equation[J]. Journal of Computational Electronics, 2017, 16 (1): 148- 154. DOI
16	SHAO S, LU T, CAI W. Adaptive conservative cell average spectral element methods for transient Wigner equation in quantum transport[J]. Communications in Computational Physics, 2011, 9 (3): 711- 739. DOI
17	SCHULZ L, SCHULZ D. Application of a slowly varying envelope function onto the analysis of the Wigner transport equation[J]. IEEE Transactions on Nanotechnology, 2016, 15 (5): 801- 809. DOI
18	LEE J H, SHIN M. Quantum transport simulation of nanowire resonant tunneling diodes based on a Wigner function model with spatially dependent effective masses[J]. IEEE Transactions on Nanotechnology, 2017, 16 (6): 1028- 1036. DOI
19	DORDA A, SCHURRER F. A WENO-solver combined with adaptive momentum discretization for the Wigner transport equation and its application to resonant tunneling diodes[J]. Journal of Computational Physics, 2015, 284, 95- 116. DOI
20	SHIFREN L, FERRY D. A Wigner function based ensemble Monte Carlo approach for accurate incorporation of quantum effects in device simulation[J]. Journal of Computational Electronics, 2002, 1 (1-2): 55- 58.
21	ELLINGHAUS P, NEDJALKOV M, SELBERHERR S. The influence of electrostatic lenses on wave packet dynamics[C]. International Conference on Large-Scale Scientific Computing, Springer, Cham, 2015: 277-284.
22	LI Gang, ZHANG Baoyin, DENG Li, SHANGGUAN Danhua, et al. Pseudo-random numbers for identical results on varying numbers of processors in domain decomposed particle Monte Carlo simulations[J]. Chinese J Comput Phys, 2017, 34 (1): 67- 72.
23	XU Haiyan, HUANG Zhengfeng, CAI Shaohui. Global variance reduction method for Monte Carlo particle transport problemes[J]. Chinese J Comput Phys, 2010, 27 (5): 722- 732.
24	HU Xiaoyan, CAO Xiaolin, GUO Hong, et al. Parallel algorithm of coupled Fourier transform and fluid numerical computation[J]. Chinese J Comput Phys, 2012, 29 (4): 484- 488.
25	ELLINGHAUS P. Two-dimensional Wigner Monte Carlo simulation for time-resolved quantum transport with scattering[D]. Vienna: Universität Wien, 2016.
26	CHEN X, LI C F, BAN Y. Novel shift in transmission through a two-dimensional semiconductor barrier[J]. Physics Letters A, 2006, 354 (1/2): 161- 165.
27	BOCQUILLON E, PARMENTIER F D, GRENIER C, et al. Electron quantum optics: Partitioning electrons one by one[J]. Physical Review Letters, 2012, 108 (19): 196803. DOI
28	ELLINGHAUS P, WEINBUB J, NEDJALKOV M, et al. Analysis of lense-governed Wigner signed particle quantum dynamics[J]. Physica Status Solidi-Rapid Research Letters, 2017, 11 (7): 1700102. DOI

[1]	徐小东, 周彬, 郭金川, 易明皓, 肖飞虎. 微结构X射线源中电子与阳极作用的蒙特卡罗模拟[J]. 计算物理, 2018, 35(1): 95-102.
[2]	李超龙, 石海泉, 吕建钦. 双圆筒加速透镜中强流束传输的模拟[J]. 计算物理, 2013, 30(3): 403-408.
[3]	王莉萍, 唐天同. 一种自动微分技术:微分代数及其在带电粒子光学中的应用[J]. 计算物理, 1999, 16(3): 225-234.
[4]	周庆, 陈尔纲, 胡宗高. 磁六极球差校正系统的计算与分析[J]. 计算物理, 1997, 14(S1): 485-486.
[5]	宋志棠, 刘纯亮, 夏慧琴. 环形电场轴向三阶色散象差系数解析表达式的计算机推导[J]. 计算物理, 1997, 14(3): 368-374.
[6]	雷威, 童林夙. 彩色显示管电子光学系统的计算和分析[J]. 计算物理, 1996, 13(3): 323-332.