球状胶束中表面活性剂脱附的离子调控机制

doi:10.19596/j.cnki.1001-246x.8701

摘要/Abstract

摘要：

建立耦合伞状采样的粗粒度分子动力学方法，研究球状胶束中表面活性剂分子的脱附过程，揭示表面活性剂聚集数、盐种类及浓度对表面活性剂脱附过程的影响机制。发现球状胶束半径及偏心率均随聚集数增加而增大，盐浓度的影响主要取决于抗衡离子的半径和吸附特性，半径更大、吸附更强的水杨酸根离子对胶束结构的影响更为显著；基于伞状采样方法获得了表面活性剂脱附自由能、脱附时间等关键参数，发现球状胶束中表面活性剂脱附自由能和脱附时间均随聚集数和盐浓度呈非单调变化，揭示其主要机制为离子吸附引起的静电屏蔽作用；发现自由能在表面活性剂脱附过程中起主导作用，结合胶束热力学理论发展了临界胶束浓度预测方法，获得了临界胶束浓度下胶束尺寸的分布范围。

关键词: 胶束, 表面活性剂脱附, 离子调控, 分子动力学, 自由能计算

Abstract:

A coarse-grained molecular dynamics method coupled with umbrella sampling is developed to study the desorption process of SDS and CTAC surfactants from spherical micelles. The influence mechanisms of aggregation number, salt species and concentrations on the desorption process of surfactant from spherical micelles are revealed. We find that the radius and eccentricity of spherical micelles both increase with aggregation number rising, and the effects of salt concentration depend on the ionic radius and adsorption characteristics of the counterions. Larger salicylate ions with stronger adsorption ability have more significant effect on the micellar structures. Based on umbrella sampling method, we obtain the desorption free energy and time of surfactants from micelles. we find that the desorption free energy and desorption time of surfactant both show a non-monotonic variation with the increase of aggregation number and salt concentration, whose mechanisms are attributed to the electrostatic shielding induced by ion adsorption on micelle surface. Moreover, we reveal that the free energy plays a leading role in surfactant desorption process. Combing the theory of micelle thermodynamics, we further develop the critical micelle concentration prediction method, and obtain the distribution range of micelle size under the critical micelle concentration of surfactants.

Key words: micelle, surfactant desorption, ionic regulation, molecular dynamics, free energy calculation

中图分类号:

O469

温伯尧, 高根英, 路熙, 关松涛, 骆政园, 白博峰. 球状胶束中表面活性剂脱附的离子调控机制[J]. 计算物理, 2024, 41(2): 193-202.

Boyao WEN, Genying GAO, Xi LU, Songtao GUAN, Zhengyuan LUO, Bofeng BAI. Ionic Regulation Mechanisms of Surfactant Desorption from the Spherical Micelles[J]. Chinese Journal of Computational Physics, 2024, 41(2): 193-202.

图/表 8

图1 球状胶束模型、分子粗粒化及Martini珠子类型

Fig.1 Spherical micelle model and coarse-grained representations of molecules and their Martini bead types

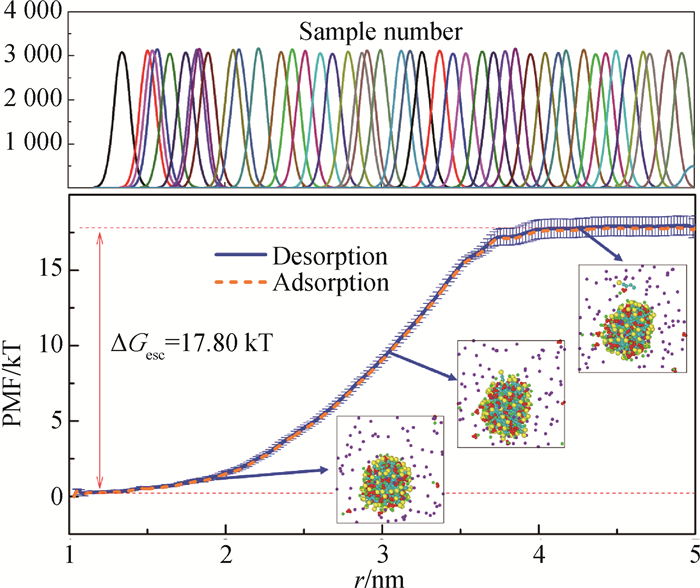
图2 球状胶束中表面活性剂吸附/脱附过程的PMF曲线及采样直方图(CTAC/NaSal体系，N = 80，R = 1.0)

Fig.2 PMF profiles and sampling histogram of surfactant adsorption and desorption processes at or from the spherical micelle (CTAC/NaSal system, N = 80, R = 1.0)

表1 SDS球状胶束结构性质(N = 60，R = 0)

Table 1 Structural properties of SDS spherical micelles (N = 60, R = 0)

	R_g/nm	R_s/nm
Our simulation results	1.55±0.05	2.00±0.06
Ref.[30]	1.55±0.30	1.98±0.27
Ref.[31]	1.50	1.96
Ref.[32]	1.58	1.90
Ref.[33]	1.64	1.99
Ref.[34]	1.60±0.06	1.97±0.08
Ref.[35]	1.54	2.00

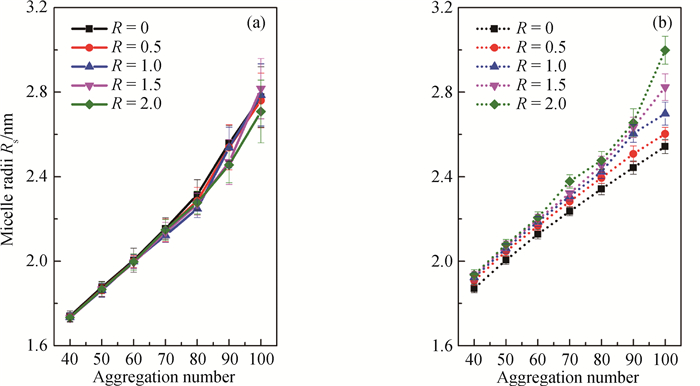
图3 不同聚集数和盐-表面活性剂浓度比的球状胶束半径 (a) SDS/NaCl体系; (b) CTAC/NaSal体系

Fig.3 Micelle radius of spherical micelle with different aggregation number and salt-to-surfactant concentration ratio (a) SDS/NaCl system; (b) CTAC/NaSal system

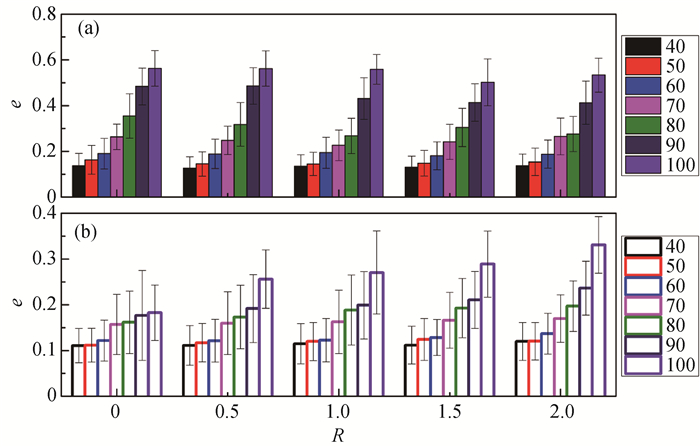
图4 不同聚集数和和盐-表面活性剂浓度比的球状胶束偏心率 (a) SDS/NaCl体系; (b) CTAC/NaSal体系

Fig.4 Eccentricities e of spherical micelles with different aggregation number and salt-to-surfactant concentration ratio (a) SDS/NaCl system; (b) CTAC/NaSal system

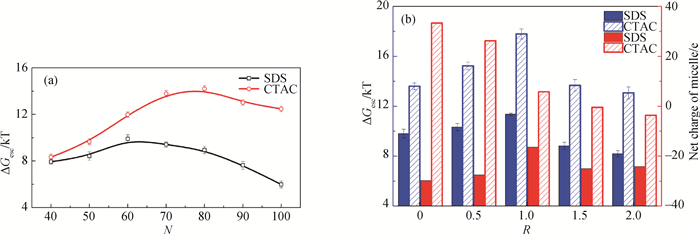
图5 不同聚集数和盐-表面活性剂浓度比的球状胶束中表面活性剂分子的脱附自由能 (a) 聚集数; (b) 盐-表面活性剂浓度比对脱附自由能及胶束净电荷值

Fig.5 Desorption free energy of surfactant from spherical micelle with different aggregation number and salt-to-surfactant concentration ratio (a) aggregation number; (b) salt-to-surfactant concentration ratio on desorption free energy and net charge of micelle

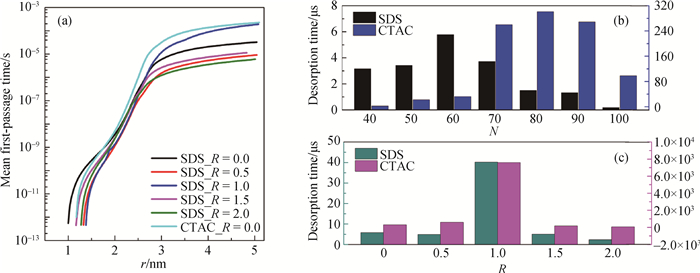
图6 球状胶束表面活性剂分子脱附过程的平均首次通过时间及脱附时间 (a) 相同聚集数下CTAC和SDS分子脱附的平均首次通过时间; (b) 不同聚集数下表面活性剂分子的脱附时间; (c) 不同盐-表面活性剂浓度比下表面活性剂分子的脱附时间

Fig.6 Mean first-passage time profiles and desorption time of SDS molecules from the interfaces (a) mean first-passage time of SDS molecules from the oil-water interfaces with different adsorbed numbers; (b) desorption time of SDS molecules from interfaces with different adsorbed numbers; (c) effects of ionic species and concentrations on the desorption time of SDS molecules

图7 不同聚集数下SDS和CTAC表面活性剂CMC预测值

Fig.7 Predicted CMC values of SDS and CTAC surfactants with different aggregation numbers

参考文献 36

1	DHAKAL S , SURESHKUMAR R . Topology, length scales, and energetics of surfactant micelles[J]. The Journal of Chemical Physics, 2015, 143 (2): 024905. DOI
2	杨少东, 叶学民, 李春曦. 活性剂浓度分布对液膜排液过程的影响[J]. 计算物理, 2018, 35 (5): 577- 586.
3	BALLAUFF M . Self-assembly creates 2D materials[J]. Science, 2016, 352 (6286): 656- 657. DOI
4	LI Qintang , WANG Jiao , LEI Nana , et al. Phase behaviours of a cationic surfactant in deep eutectic solvents: From micelles to lyotropic liquid crystals[J]. Physical Chemistry Chemical Physics, 2018, 20 (17): 12175- 12181. DOI
5	王雯婷, 尹慧瑾, 潘俊星, 等. 光掩膜诱导的聚合物/纳米粒子共混体系的自组装计算机模拟[J]. 计算物理, 2022, 39 (5): 598- 608.
6	LARSSON J , SANCHEZ-FERNANDEZ A , LEUNG A E , et al. Molecular structure of maltoside surfactants controls micelle formation and rheological behavior[J]. Journal of Colloid and Interface Science, 2021, 581 (Pt B): 895- 904.
7	SAMBASIVAM A , SANGWAI A V , SURESHKUMAR R . Dynamics and scission of rodlike cationic surfactant micelles in shear flow[J]. Physical Review Letters, 2015, 114 (15): 158302. DOI
8	AMANN M , WILLNER L , STELLBRINK J , et al. Studying the concentration dependence of the aggregation number of a micellar model system by SANS[J]. Soft Matter, 2015, 11 (21): 4208- 4217. DOI
9	ZANA R . Dynamics of surfactant self-assemblies: Micelles, microemulsions, vesicles and lyotropic phases[M]. Boca Raton: CRC Press, 2005.
10	PATIST A , KANICKY J R , SHUKLA P K , et al. Importance of micellar kinetics in relation to technological processes[J]. Journal of Colloid and Interface Science, 2002, 245 (1): 1- 15. DOI
11	OH S G , SHAH D O . Micellar lifetime: Its relevance to various technological processes[J]. Journal of Dispersion Science and Technology, 1994, 15 (3): 297- 316. DOI
12	SHCHEKIN A K , ADZHEMYAN L T , BABINTSEV I A , et al. Kinetics of aggregation and relaxation in micellar surfactant solutions[J]. Colloid Journal, 2018, 80 (2): 107- 140. DOI
13	WATON G . Kinetics associated with the change of the number density of micelles in solution[J]. The Journal of Physical Chemistry B, 1997, 101 (47): 9727- 9731. DOI
14	ANIANSSON E A G , WALL S N . Kinetics of step-wise micelle association[J]. The Journal of Physical Chemistry, 1974, 78 (10): 1024- 1030. DOI
15	BECKER R , DÖRING W . Kinetische behandlung der keimbildung in übersättigten dämpfen[J]. Annalen der Physik, 1935, 416 (8): 719- 752. DOI
16	SHCHEKIN A K , BABINTSEV I A , ADZHEMYAN L T . Full-time kinetics of self-assembly and disassembly in micellar solution via the generalized Smoluchowski equation with fusion and fission of surfactant aggregates[J]. The Journal of Chemical Physics, 2016, 145 (17): 174105. DOI
17	史晓蕊, 刘振宇, 吴慧英. 纳米孔壁面作用对蛋白质过孔影响的粗粒化分子动力学模拟[J]. 计算物理, 2020, 37 (1): 63- 68.
18	SAMMALKORPI M , KARTTUNEN M , HAATAJA M . Micelle fission through surface instability and formation of an interdigitating stalk[J]. Journal of the American Chemical Society, 2008, 130 (52): 17977- 17980. DOI
19	曹仁义, 黄涛, 程林松, 等. 水驱油藏中原油极性物质对吸附和润湿性影响的分子模拟[J]. 计算物理, 2021, 38 (5): 595- 602.
20	俞宏伟, 李实, 李金龙, 等. 气驱油油气混相过程的界面传质特性及其分子机制[J]. 物理化学学报, 2022, 38 (5): 2006061.
21	BROCOS P , MENDOZA-ESPINOSA P , CASTILLO R , et al. Multiscale molecular dynamics simulations of micelles: Coarse-grain for self-assembly and atomic resolution for finer details[J]. Soft Matter, 2012, 8 (34): 9005- 9014. DOI
22	JUSUFI A , PANAGIOTOPOULOS A Z . Explicit- and implicit-solvent simulations of micellization in surfactant solutions[J]. Langmuir, 2015, 31 (11): 3283- 3292. DOI
23	YUAN Fang , WANG Shihu , LARSON R G . Potentials of mean force and escape times of surfactants from micelles and hydrophobic surfaces using molecular dynamics simulations[J]. Langmuir, 2015, 31 (4): 1336- 1343. DOI
24	WEN Boyao , BAI Bofeng , LARSON R G . Surfactant desorption and scission free energies for cylindrical and spherical micelles from umbrella-sampling molecular dynamics simulations[J]. Journal of Colloid and Interface Science, 2021, 599, 773- 784. DOI
25	温伯尧, 杨海中, 姚秀田, 等. 油水界面上表面活性剂的脱附动力学及其离子调控[J]. 科学通报, 2022, 67 (25): 3088- 3096.
26	SANGWAI A V , SURESHKUMAR R . Coarse-grained molecular dynamics simulations of the sphere to rod transition in surfactant micelles[J]. Langmuir, 2011, 27 (11): 6628- 6638. DOI
27	RUSSO KRAUSS I , CAVASSO D , CICCARELLI D , et al. A hofmeister series perspective on the mixed micellization of cationic and non-ionic surfactants[J]. Journal of Molecular Liquids, 2021, 335, 116205. DOI
28	SOUZA P C T , ALESSANDRI R , BARNOUD J , et al. Martini 3: A general purpose force field for coarse-grained molecular dynamics[J]. Nature Methods, 2021, 18 (4): 382- 388. DOI
29	GROSSFIELD A. WHAM: The weighted histogram analysis method, version 2.0[Z]. 2018.
30	WANG Shihu , LARSON R G . Coarse-grained molecular dynamics simulation of self-assembly and surface adsorption of ionic surfactants using an implicit water model[J]. Langmuir, 2015, 31 (4): 1262- 1271. DOI
31	PALAZZESI F , CALVARESI M , ZERBETTO F . A molecular dynamics investigation of structure and dynamics of SDS and SDBS micelles[J]. Soft Matter, 2011, 7 (19): 9148- 9156. DOI
32	SHANG B Z , WANG Zuowei , LARSON R G . Molecular dynamics simulation of interactions between a sodium dodecyl sulfate micelle and a poly(ethylene oxide) polymer[J]. The Journal of Physical Chemistry B, 2008, 112 (10): 2888- 2900. DOI
33	RAKITIN A R , PACK G R . Molecular dynamics simulations of ionic interactions with dodecyl sulfate micelles[J]. The Journal of Physical Chemistry B, 2004, 108 (8): 2712- 2716. DOI
34	MACKERELL A D Jr . Molecular dynamics simulation analysis of a sodium dodecyl sulfate micelle in aqueous solution: Decreased fluidity of the micelle hydrocarbon interior[J]. The Journal of Physical Chemistry, 1995, 99 (7): 1846- 1855. DOI
35	ITRI R , AMARAL L Q . Distance distribution function of sodium dodecyl sulfate micelles by x-ray scattering[J]. The Journal of Physical Chemistry, 1991, 95 (1): 423- 427. DOI
36	TANG Xueming , KOENIG P H , LARSON R G . Molecular dynamics simulations of sodium dodecyl sulfate micelles in water-the effect of the force field[J]. The Journal of Physical Chemistry B, 2014, 118 (14): 3864- 3880. DOI

[1]	高旭东, 孙淑义, 魏雯静, 李公平. 金红石TiO₂辐照损伤模拟研究[J]. 计算物理, 2024, 41(2): 214-221.
[2]	李禹, 刘慧卿, 冯亚斌, 东晓虎, 王庆, 张波. 典型表活剂与稠油在蒙脱石表面吸附行为的分子动力学模拟[J]. 计算物理, 2023, 40(5): 583-596.
[3]	韦昭召, 刘凯, 李会军. NiAl合金纳米线弯曲形变行为的分子动力学模拟[J]. 计算物理, 2023, 40(4): 425-435.
[4]	侯兆阳, 牛媛, 肖启鑫, 王真, 邓庆田. Al纳米线不同晶向力学行为和变形机制的模拟[J]. 计算物理, 2022, 39(3): 341-351.
[5]	王晓慧, 张平. 高压下FCC相金属氢结构稳定性和非谐效应的理论研究[J]. 计算物理, 2022, 39(2): 159-164.
[6]	阿湖宝, 杨志兵, 胡冉, 陈益峰. 纳米尺度下毛细流动的分子动力学模拟[J]. 计算物理, 2021, 38(5): 603-611.
[7]	王国华, 崔雅茹, 杨泽, 李小明, 汤宏亮, 杨树峰. Fe_xO-SiO₂-CaO-MgO-“NiO”系镍渣势函数及分子动力学模拟[J]. 计算物理, 2021, 38(2): 215-223.
[8]	王雪梅, 董斌, 朱子亮, 杨俊升. 聚合物分子与官能化纳米管相互作用及扩散特性的分子动力学模拟[J]. 计算物理, 2020, 37(5): 589-594.
[9]	和二斌, 罗志荣, 朱留华. 肌红蛋白力致去折叠的全原子分析[J]. 计算物理, 2020, 37(2): 205-211.
[10]	周璐, 马红和. 超临界水中硫酸钠结晶动力学的分子动力学模拟[J]. 计算物理, 2020, 37(2): 212-220.
[11]	史晓蕊, 刘振宇, 吴慧英. 纳米孔壁面作用对蛋白质过孔影响的粗粒化分子动力学模拟[J]. 计算物理, 2020, 37(1): 63-68.
[12]	柴汝宽, 刘月田, 王俊强, 辛晶, 皮建, 李长勇. 分子动力学模拟方解石和白云石润湿性[J]. 计算物理, 2019, 36(4): 474-482.
[13]	王帅创, 张弓木, 孙博, 宋海峰, 田明锋, 方俊, 刘海风. 量子分子动力学模拟液体钚的输运性质[J]. 计算物理, 2019, 36(3): 253-258.
[14]	梁华, 李茂生. 孔洞和空位对单晶铝力学性能影响的分子动力学研究[J]. 计算物理, 2019, 36(2): 211-218.
[15]	张海燕, 殷新春. 简单金属固液界面固化过程生长机制的分子动力学研究[J]. 计算物理, 2019, 36(1): 80-88.